Hagen–Poiseuille Equation on:
[Wikipedia]
[Google]
[Amazon]
In nonideal
 Assume the liquid exhibits
Assume the liquid exhibits
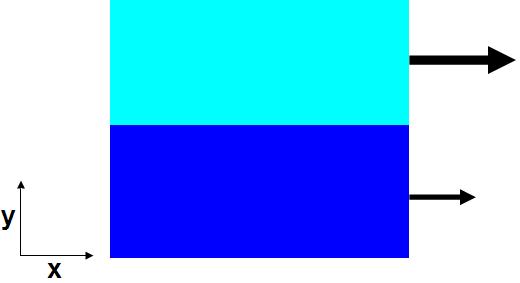 When two layers of liquid in contact with each other move at different speeds, there will be a
When two layers of liquid in contact with each other move at different speeds, there will be a
Poiseuille's law for power-law non-Newtonian fluidHagen–Poiseuille equation calculator
{{DEFAULTSORT:Hagen-Poiseuille equation Equations of fluid dynamics Mathematics in medicine
fluid dynamics
In physics and engineering, fluid dynamics is a subdiscipline of fluid mechanics that describes the flow of fluids— liquids and gases. It has several subdisciplines, including ''aerodynamics'' (the study of air and other gases in motion) an ...
, the Hagen–Poiseuille equation, also known as the Hagen–Poiseuille law, Poiseuille law or Poiseuille equation, is a physical law
Scientific laws or laws of science are statements, based on repeated experiments or observations, that describe or predict a range of natural phenomena. The term ''law'' has diverse usage in many cases (approximate, accurate, broad, or narrow) a ...
that gives the pressure drop
Pressure drop is defined as the difference in total pressure between two points of a fluid carrying network. A pressure drop occurs when frictional forces, caused by the resistance to flow, act on a fluid as it flows through the tube. The main de ...
in an incompressible
In fluid mechanics or more generally continuum mechanics, incompressible flow ( isochoric flow) refers to a flow in which the material density is constant within a fluid parcel—an infinitesimal volume that moves with the flow velocity. An eq ...
and Newtonian fluid in laminar flow
In fluid dynamics, laminar flow is characterized by fluid particles following smooth paths in layers, with each layer moving smoothly past the adjacent layers with little or no mixing. At low velocities, the fluid tends to flow without lateral mi ...
flowing through a long cylindrical pipe of constant cross section.
It can be successfully applied to air flow in lung
The lungs are the primary organs of the respiratory system in humans and most other animals, including some snails and a small number of fish. In mammals and most other vertebrates, two lungs are located near the backbone on either side of t ...
alveoli Alveolus (; pl. alveoli, adj. alveolar) is a general anatomical term for a concave cavity or pit.
Uses in anatomy and zoology
* Pulmonary alveolus, an air sac in the lungs
** Alveolar cell or pneumocyte
** Alveolar duct
** Alveolar macrophage
* ...
, or the flow through a drinking straw or through a hypodermic needle
A hypodermic needle (from Greek ὑπο- (''hypo-'' = under), and δέρμα (''derma'' = skin)), one of a category of medical tools which enter the skin, called sharps, is a very thin, hollow tube with one sharp tip. It is commonly used w ...
. It was experimentally derived independently by Jean Léonard Marie Poiseuille
Jean Léonard Marie Poiseuille (; 22 April 1797 – 26 December 1869) was a French physicist and physiologist.
Poiseuille was born in Paris, France, and he died there on 26 December 1869.
Fluid flow
From 1815 to 1816 he studied at the École Po ...
in 1838 and Gotthilf Heinrich Ludwig Hagen
Gotthilf Heinrich Ludwig Hagen (3 March 1797 – 3 February 1884) was a German civil engineer who made important contributions to fluid dynamics, hydraulic engineering and probability theory.
Life and work
Hagen was born in Königsberg, East P ...
, and published by Poiseuille in 1840–41 and 1846. The theoretical justification of the Poiseuille law was given by George Stokes in 1845.
The assumptions of the equation are that the fluid is incompressible
In fluid mechanics or more generally continuum mechanics, incompressible flow ( isochoric flow) refers to a flow in which the material density is constant within a fluid parcel—an infinitesimal volume that moves with the flow velocity. An eq ...
and Newtonian; the flow is laminar through a pipe of constant circular cross-section that is substantially longer than its diameter; and there is no acceleration
In mechanics, acceleration is the rate of change of the velocity of an object with respect to time. Accelerations are vector quantities (in that they have magnitude and direction). The orientation of an object's acceleration is given by the ...
of fluid in the pipe. For velocities and pipe diameters above a threshold, actual fluid flow is not laminar but turbulent
In fluid dynamics, turbulence or turbulent flow is fluid motion characterized by chaotic changes in pressure and flow velocity. It is in contrast to a laminar flow, which occurs when a fluid flows in parallel layers, with no disruption between t ...
, leading to larger pressure drops than calculated by the Hagen–Poiseuille equation.
Poiseuille's equation describes the pressure drop ''due to'' the viscosity of the fluid; other types of pressure drops may still occur in a fluid (see a demonstration here). For example, the pressure needed to drive a viscous fluid up against gravity would contain both that as needed in Poiseuille's law ''plus'' that as needed in Bernoulli's equation
In fluid dynamics, Bernoulli's principle states that an increase in the speed of a fluid occurs simultaneously with a decrease in static pressure or a decrease in the fluid's potential energy. The principle is named after the Swiss mathematic ...
, such that any point in the flow would have a pressure greater than zero (otherwise no flow would happen).
Another example is when blood flows into a narrower constriction
Constriction is a method used by various snake species to kill or subdue their prey. Although some species of venomous and mildly venomous snakes do use constriction to subdue their prey, most snakes which use constriction lack venom. The snake i ...
, its speed will be greater than in a larger diameter (due to continuity of volumetric flow rate
In physics and engineering, in particular fluid dynamics, the volumetric flow rate (also known as volume flow rate, or volume velocity) is the volume of fluid which passes per unit time; usually it is represented by the symbol (sometimes ). I ...
), and its pressure will be lower than in a larger diameter (due to Bernoulli's equation). However, the viscosity of blood will cause ''additional'' pressure drop along the direction of flow, which is proportional to length traveled (as per Poiseuille's law). Both effects contribute to the ''actual'' pressure drop.
Equation
In standard fluid-kinetics notation: : where: * is the pressure difference between the two ends, * is the length of pipe, * is thedynamic viscosity
The viscosity of a fluid is a measure of its resistance to deformation at a given rate. For liquids, it corresponds to the informal concept of "thickness": for example, syrup has a higher viscosity than water.
Viscosity quantifies the inter ...
,
* is the volumetric flow rate
In physics and engineering, in particular fluid dynamics, the volumetric flow rate (also known as volume flow rate, or volume velocity) is the volume of fluid which passes per unit time; usually it is represented by the symbol (sometimes ). I ...
,
* is the pipe radius
In classical geometry, a radius ( : radii) of a circle or sphere is any of the line segments from its center to its perimeter, and in more modern usage, it is also their length. The name comes from the latin ''radius'', meaning ray but also the ...
,
* is the cross sectional area
In geometry and science, a cross section is the non-empty intersection of a solid body in three-dimensional space with a plane, or the analog in higher- dimensional spaces. Cutting an object into slices creates many parallel cross-sections. T ...
of pipe.
The equation does not hold close to the pipe entrance.
The equation fails in the limit of low viscosity, wide and/or short pipe. Low viscosity or a wide pipe may result in turbulent flow, making it necessary to use more complex models, such as the Darcy–Weisbach equation
In fluid dynamics, the Darcy–Weisbach equation is an empirical equation that relates the head loss, or pressure loss, due to friction along a given length of pipe to the average velocity of the fluid flow for an incompressible fluid. The equation ...
. The ratio of length to radius of a pipe should be greater than one forty-eighth of the Reynolds number
In fluid mechanics, the Reynolds number () is a dimensionless quantity that helps predict fluid flow patterns in different situations by measuring the ratio between inertial and viscous forces. At low Reynolds numbers, flows tend to be domi ...
for the Hagen–Poiseuille law to be valid. If the pipe is too short, the Hagen–Poiseuille equation may result in unphysically high flow rates; the flow is bounded by Bernoulli's principle
In fluid dynamics, Bernoulli's principle states that an increase in the speed of a fluid occurs simultaneously with a decrease in static pressure or a decrease in the fluid's potential energy. The principle is named after the Swiss mathematici ...
, under less restrictive conditions, by
:
because it is impossible to have negative (absolute) pressure (not to be confused with gauge pressure
Pressure measurement is the measurement of an applied force by a fluid (liquid or gas) on a surface. Pressure is typically measured in units of force per unit of surface area. Many techniques have been developed for the measurement of pressur ...
) in an incompressible flow.
Relation to the Darcy–Weisbach equation
Normally, Hagen–Poiseuille flow implies not just the relation for the pressure drop, above, but also the full solution for the laminar flow profile, which is parabolic. However, the result for the pressure drop can be extended to turbulent flow by inferring an effective turbulent viscosity in the case of turbulent flow, even though the flow profile in turbulent flow is strictly speaking not actually parabolic. In both cases, laminar or turbulent, the pressure drop is related to the stress at the wall, which determines the so-called friction factor. The wall stress can be determined phenomenologically by theDarcy–Weisbach equation
In fluid dynamics, the Darcy–Weisbach equation is an empirical equation that relates the head loss, or pressure loss, due to friction along a given length of pipe to the average velocity of the fluid flow for an incompressible fluid. The equation ...
in the field of hydraulics
Hydraulics (from Greek: Υδραυλική) is a technology and applied science using engineering, chemistry, and other sciences involving the mechanical properties and use of liquids. At a very basic level, hydraulics is the liquid counter ...
, given a relationship for the friction factor in terms of the Reynolds number. In the case of laminar flow, for a circular cross section:
:
where is the Reynolds number
In fluid mechanics, the Reynolds number () is a dimensionless quantity that helps predict fluid flow patterns in different situations by measuring the ratio between inertial and viscous forces. At low Reynolds numbers, flows tend to be domi ...
, is the fluid density, and is the mean flow velocity, which is half the maximal flow velocity in the case of laminar flow. It proves more useful to define the Reynolds number in terms of the mean flow velocity because this quantity remains well defined even in the case of turbulent flow, whereas the maximal flow velocity may not be, or in any case, it may be difficult to infer. In this form the law approximates the ''Darcy friction factor
Darcy, Darci or Darcey may refer to:
Science
* Darcy's law, which describes the flow of a fluid through porous material
* Darcy (unit), a unit of permeability of fluids in porous material
* Darcy friction factor in the field of fluid mechanics
* ...
'', the ''energy (head) loss factor'', ''friction loss factor'' or ''Darcy (friction) factor'' in the laminar flow at very low velocities in cylindrical tube. The theoretical derivation of a slightly different form of the law was made independently by Wiedman in 1856 and Neumann and E. Hagenbach in 1858 (1859, 1860). Hagenbach was the first who called this law Poiseuille's law.
The law is also very important in hemorheology
Hemorheology, also spelled haemorheology (from Greek ‘αἷμα, ''haima'' 'blood' and rheology, from Greek ῥέω ''rhéō'', 'flow' and -λoγία, ''-logia'' 'study of'), or blood rheology, is the study of flow properties of blood and its ...
and hemodynamics
Hemodynamics or haemodynamics are the dynamics of blood flow. The circulatory system is controlled by homeostatic mechanisms of autoregulation, just as hydraulic circuits are controlled by control systems. The hemodynamic response continuously m ...
, both fields of physiology
Physiology (; ) is the scientific study of functions and mechanisms in a living system. As a sub-discipline of biology, physiology focuses on how organisms, organ systems, individual organs, cells, and biomolecules carry out the chemical ...
.
Poiseuille's law was later in 1891 extended to turbulent flow
In fluid dynamics, turbulence or turbulent flow is fluid motion characterized by chaotic changes in pressure and flow velocity. It is in contrast to a laminar flow, which occurs when a fluid flows in parallel layers, with no disruption between ...
by L. R. Wilberforce, based on Hagenbach's work.
Derivation
The Hagen–Poiseuille equation can be derived from theNavier–Stokes equations
In physics, the Navier–Stokes equations ( ) are partial differential equations which describe the motion of viscous fluid substances, named after French engineer and physicist Claude-Louis Navier and Anglo-Irish physicist and mathematician Geo ...
. The laminar flow
In fluid dynamics, laminar flow is characterized by fluid particles following smooth paths in layers, with each layer moving smoothly past the adjacent layers with little or no mixing. At low velocities, the fluid tends to flow without lateral mi ...
through a pipe of uniform (circular) cross-section is known as Hagen–Poiseuille flow. The equations governing the Hagen–Poiseuille flow can be derived directly from the Navier–Stokes momentum equations in 3D cylindrical coordinates by making the following set of assumptions:
# The flow is steady ( ).
# The radial and azimuthal components of the fluid velocity are zero ( ).
# The flow is axisymmetric ( ).
# The flow is fully developed ( ). Here However, this can be proved via mass conservation, and the above assumptions.
Then the angular equation in the momentum equations and the continuity equation
A continuity equation or transport equation is an equation that describes the transport of some quantity. It is particularly simple and powerful when applied to a conserved quantity, but it can be generalized to apply to any extensive quantity. S ...
are identically satisfied. The radial momentum equation reduces to , i.e., the pressure
Pressure (symbol: ''p'' or ''P'') is the force applied perpendicular to the surface of an object per unit area over which that force is distributed. Gauge pressure (also spelled ''gage'' pressure)The preferred spelling varies by country and e ...
is a function of the axial coordinate only. For brevity, use instead of . The axial momentum equation reduces to
:
where is the dynamic viscosity of the fluid. In the above equation, the left-hand side is only a function of and the right-hand side term is only a function of , implying that both terms must be the same constant. Evaluating this constant is straightforward. If we take the length of the pipe to be and denote the pressure difference between the two ends of the pipe by (high pressure minus low pressure), then the constant is simply
:
defined such that is positive. The solution is
:
Since needs to be finite at , . The no slip boundary condition
In mathematics, in the field of differential equations, a boundary value problem is a differential equation together with a set of additional constraints, called the boundary conditions. A solution to a boundary value problem is a solution to th ...
at the pipe wall requires that at (radius of the pipe), which yields . Thus we have finally the following parabolic velocity
Velocity is the directional speed of an object in motion as an indication of its rate of change in position as observed from a particular frame of reference and as measured by a particular standard of time (e.g. northbound). Velocity is a ...
profile:
:
The maximum velocity occurs at the pipe centerline (), . The average velocity can be obtained by integrating over the pipe cross section
Cross section may refer to:
* Cross section (geometry)
** Cross-sectional views in architecture & engineering 3D
*Cross section (geology)
* Cross section (electronics)
* Radar cross section, measure of detectability
* Cross section (physics)
**Abs ...
,
:
The easily measurable quantity in experiments is the volumetric flow rate . Rearrangement of this gives the Hagen–Poiseuille equation
:
Although more lengthy than directly using the Navier–Stokes equations
In physics, the Navier–Stokes equations ( ) are partial differential equations which describe the motion of viscous fluid substances, named after French engineer and physicist Claude-Louis Navier and Anglo-Irish physicist and mathematician Geo ...
, an alternative method of deriving the Hagen–Poiseuille equation is as follows.
Liquid flow through a pipe
laminar flow
In fluid dynamics, laminar flow is characterized by fluid particles following smooth paths in layers, with each layer moving smoothly past the adjacent layers with little or no mixing. At low velocities, the fluid tends to flow without lateral mi ...
. Laminar flow in a round pipe prescribes that there are a bunch of circular layers (lamina) of liquid, each having a velocity determined only by their radial distance from the center of the tube. Also assume the center is moving fastest while the liquid touching the walls of the tube is stationary (due to the no-slip condition
In fluid dynamics, the no-slip condition for viscous fluids assumes that at a solid boundary, the fluid will have zero velocity relative to the boundary.
The fluid velocity at all fluid–solid boundaries is equal to that of the solid boundary. C ...
).
To figure out the motion of the liquid, all forces acting on each lamina must be known:
# The pressure
Pressure (symbol: ''p'' or ''P'') is the force applied perpendicular to the surface of an object per unit area over which that force is distributed. Gauge pressure (also spelled ''gage'' pressure)The preferred spelling varies by country and e ...
force pushing the liquid through the tube is the change in pressure multiplied by the area: . This force is in the direction of the motion of the liquid. The negative sign comes from the conventional way we define .
# Viscosity
The viscosity of a fluid is a measure of its resistance to deformation at a given rate. For liquids, it corresponds to the informal concept of "thickness": for example, syrup has a higher viscosity than water.
Viscosity quantifies the inte ...
effects will pull from the faster lamina immediately closer to the center of the tube.
# Viscosity
The viscosity of a fluid is a measure of its resistance to deformation at a given rate. For liquids, it corresponds to the informal concept of "thickness": for example, syrup has a higher viscosity than water.
Viscosity quantifies the inte ...
effects will drag from the slower lamina immediately closer to the walls of the tube.
Viscosity
 When two layers of liquid in contact with each other move at different speeds, there will be a
When two layers of liquid in contact with each other move at different speeds, there will be a shear force
In solid mechanics, shearing forces are unaligned forces acting on one part of a body in a specific direction, and another part of the body in the opposite direction. When the forces are collinear (aligned with each other), they are called t ...
between them. This force is proportional to the area
Area is the quantity that expresses the extent of a region on the plane or on a curved surface. The area of a plane region or ''plane area'' refers to the area of a shape
A shape or figure is a graphics, graphical representation of an obje ...
of contact , the velocity gradient perpendicular to the direction of flow , and a proportionality constant (viscosity) and is given by
:
The negative sign is in there because we are concerned with the faster moving liquid (top in figure), which is being slowed by the slower liquid (bottom in figure). By Newton's third law of motion
Newton's laws of motion are three basic Scientific law, laws of classical mechanics that describe the relationship between the motion of an object and the forces acting on it. These laws can be paraphrased as follows:
# A body remains at re ...
, the force on the slower liquid is equal and opposite (no negative sign) to the force on the faster liquid. This equation assumes that the area of contact is so large that we can ignore any effects from the edges and that the fluids behave as Newtonian fluid
A Newtonian fluid is a fluid in which the viscous stresses arising from its flow are at every point linearly correlated to the local strain rate — the rate of change of its deformation over time. Stresses are proportional to the rate of chang ...
s.
Faster lamina
Assume that we are figuring out the force on the lamina withradius
In classical geometry, a radius ( : radii) of a circle or sphere is any of the line segments from its center to its perimeter, and in more modern usage, it is also their length. The name comes from the latin ''radius'', meaning ray but also the ...
. From the equation above, we need to know the area
Area is the quantity that expresses the extent of a region on the plane or on a curved surface. The area of a plane region or ''plane area'' refers to the area of a shape
A shape or figure is a graphics, graphical representation of an obje ...
of contact and the velocity gradient
In vector calculus, the gradient of a scalar-valued differentiable function of several variables is the vector field (or vector-valued function) \nabla f whose value at a point p is the "direction and rate of fastest increase". If the gradi ...
. Think of the lamina as a ring of radius , thickness , and length . The area of contact between the lamina and the faster one is simply the surface area of the cylinder: . We don't know the exact form for the velocity of the liquid within the tube yet, but we do know (from our assumption above) that it is dependent on the radius. Therefore, the velocity gradient is the change of the velocity with respect to the change in the radius at the intersection of these two laminae. That intersection is at a radius of . So, considering that this force will be positive with respect to the movement of the liquid (but the derivative of the velocity is negative), the final form of the equation becomes
:
where the vertical bar and subscript following the derivative
In mathematics, the derivative of a function of a real variable measures the sensitivity to change of the function value (output value) with respect to a change in its argument (input value). Derivatives are a fundamental tool of calculus. F ...
indicates that it should be taken at a radius of .
Slower lamina
Next let's find the force of drag from the slower lamina. We need to calculate the same values that we did for the force from the faster lamina. In this case, the area of contact is at instead of . Also, we need to remember that this force opposes the direction of movement of the liquid and will therefore be negative (and that the derivative of the velocity is negative). :Putting it all together
To find the solution for the flow of a laminar layer through a tube, we need to make one last assumption. There is noacceleration
In mechanics, acceleration is the rate of change of the velocity of an object with respect to time. Accelerations are vector quantities (in that they have magnitude and direction). The orientation of an object's acceleration is given by the ...
of liquid in the pipe, and by Newton's first law
Newton's laws of motion are three basic laws of classical mechanics that describe the relationship between the motion of an object and the forces acting on it. These laws can be paraphrased as follows:
# A body remains at rest, or in motion ...
, there is no net force. If there is no net force then we can add all of the forces together to get zero
:
or
:
First, to get everything happening at the same point, use the first two terms of a Taylor series expansion
In mathematics, the Taylor series or Taylor expansion of a function is an infinite sum of terms that are expressed in terms of the function's derivatives at a single point. For most common functions, the function and the sum of its Taylor serie ...
of the velocity gradient:
:
The expression is valid for all laminae. Grouping like terms and dropping the vertical bar since all derivatives are assumed to be at radius ,
:
Finally, put this expression in the form of a differential equation
In mathematics, a differential equation is an equation that relates one or more unknown functions and their derivatives. In applications, the functions generally represent physical quantities, the derivatives represent their rates of change, an ...
, dropping the term quadratic in .
:
The above equation is the same as the one obtained from the Navier–Stokes equations and the derivation from here on follows as before.
Startup of Poiseuille flow in a pipe
When a ''constant'' pressure gradient is applied between two ends of a long pipe, the flow will not immediately obtain Poiseuille profile, rather it develops through time and reaches the Poiseuille profile at steady state. TheNavier–Stokes equations
In physics, the Navier–Stokes equations ( ) are partial differential equations which describe the motion of viscous fluid substances, named after French engineer and physicist Claude-Louis Navier and Anglo-Irish physicist and mathematician Geo ...
reduce to
:
with initial and boundary conditions,
:
The velocity distribution is given by
:
where is the Bessel function of the first kind
Bessel functions, first defined by the mathematician Daniel Bernoulli and then generalized by Friedrich Bessel, are canonical solutions of Bessel's differential equation
x^2 \frac + x \frac + \left(x^2 - \alpha^2 \right)y = 0
for an arbitrary ...
of order zero and are the positive roots of this function and is the Bessel function of the first kind
Bessel functions, first defined by the mathematician Daniel Bernoulli and then generalized by Friedrich Bessel, are canonical solutions of Bessel's differential equation
x^2 \frac + x \frac + \left(x^2 - \alpha^2 \right)y = 0
for an arbitrary ...
of order one. As , Poiseuille solution is recovered.
Poiseuille flow in an annular section
If is the inner cylinder radii and is the outer cylinder radii, with ''constant'' applied pressure gradient between the two ends , the velocity distribution and the volume flux through the annular pipe are : When , , the original problem is recovered.Poiseuille flow in a pipe with an oscillating pressure gradient
Flow through pipes with an oscillating pressure gradient finds applications in blood flow through large arteries. The imposed pressure gradient is given by : where , and are constants and is the frequency. The velocity field is given by : where : where and are theKelvin functions
In applied mathematics, the Kelvin functions ber''ν''(''x'') and bei''ν''(''x'') are the real and imaginary parts, respectively, of
:J_\nu \left (x e^ \right ),\,
where ''x'' is real, and , is the ''ν''th order Bessel function of the first kin ...
and .
Plane Poiseuille flow
Plane Poiseuille flow is flow created between two infinitely long parallel plates, separated by a distance with a constant pressure gradient is applied in the direction of flow. The flow is essentially unidirectional because of infinite length. TheNavier–Stokes equations
In physics, the Navier–Stokes equations ( ) are partial differential equations which describe the motion of viscous fluid substances, named after French engineer and physicist Claude-Louis Navier and Anglo-Irish physicist and mathematician Geo ...
reduce to
:
with no-slip condition
In fluid dynamics, the no-slip condition for viscous fluids assumes that at a solid boundary, the fluid will have zero velocity relative to the boundary.
The fluid velocity at all fluid–solid boundaries is equal to that of the solid boundary. C ...
on both walls
:
Therefore, the velocity distribution and the volume flow rate per unit length are
:
Poiseuille flow through some non-circular cross-sections
Joseph Boussinesq
Joseph Valentin Boussinesq (; 13 March 1842 – 19 February 1929) was a French mathematician and physicist who made significant contributions to the theory of hydrodynamics, vibration, light, and heat.
Biography
From 1872 to 1886, he was appoi ...
derived the velocity profile and volume flow rate in 1868 for rectangular channel and tubes of equilateral triangular cross-section and for elliptical cross-section. Joseph Proudman
Joseph Proudman (30 December 1888 – 26 June 1975), CBE, FRS was a distinguished British mathematician and oceanographer of international repute. His theoretical studies into the oceanic tides not only "solved practically all the remaining ...
derived the same for isosceles triangles in 1914. Let be the constant pressure gradient acting in direction parallel to the motion.
The velocity and the volume flow rate in a rectangular channel of height and width are
:
The velocity and the volume flow rate of tube with equilateral triangular cross-section of side length are
:
The velocity and the volume flow rate in the right-angled isosceles triangle , are
:
The velocity distribution for tubes of elliptical cross-section with semiaxes and is
:
Here, when , Poiseuille flow for circular pipe is recovered and when , ''plane Poiseuille'' flow is recovered. More explicit solutions with cross-sections such as snail-shaped sections, sections having the shape of a notch circle following a semicircle, annular sections between homofocal ellipses, annular sections between non-concentric circles are also available, as reviewed by .
Poiseuille flow through arbitrary cross-section
The flow through arbitrary cross-section satisfies the condition that on the walls. The governing equation reduces to : If we introduce a new dependent variable as : then it is easy to see that the problem reduces to that integrating aLaplace equation
In mathematics and physics, Laplace's equation is a second-order partial differential equation named after Pierre-Simon Laplace, who first studied its properties. This is often written as
\nabla^2\! f = 0 or \Delta f = 0,
where \Delta = \nab ...
:
satisfying the condition
:
on the wall.
Poiseuille's equation for an ideal isothermal gas
For a compressible fluid in a tube thevolumetric flow rate
In physics and engineering, in particular fluid dynamics, the volumetric flow rate (also known as volume flow rate, or volume velocity) is the volume of fluid which passes per unit time; usually it is represented by the symbol (sometimes ). I ...
(but not the mass flow rate) and the axial velocity are not constant along the tube. The flow is usually expressed at outlet pressure. As fluid is compressed or expanded, work is done and the fluid is heated or cooled. This means that the flow rate depends on the heat transfer to and from the fluid. For an ideal gas
An ideal gas is a theoretical gas composed of many randomly moving point particles that are not subject to interparticle interactions. The ideal gas concept is useful because it obeys the ideal gas law, a simplified equation of state, and is a ...
in the isothermal
In thermodynamics, an isothermal process is a type of thermodynamic process in which the temperature ''T'' of a system remains constant: Δ''T'' = 0. This typically occurs when a system is in contact with an outside thermal reservoir, and a ...
case, where the temperature of the fluid is permitted to equilibrate with its surroundings, an approximate relation for the pressure drop can be derived. Using ideal gas equation of state for constant temperature process, the relation can be obtained. Over a short section of the pipe, the gas flowing through the pipe can be assumed to be incompressible so that Poiseuille law can be used locally,
:
Here we assumed the local pressure gradient is not too great to have any compressibility effects. Though locally we ignored the effects of pressure variation due to density variation, over long distances these effects are taken into account. Since is independent of pressure, the above equation can be integrated over the length to give
:
Hence the volumetric flow rate at the pipe outlet is given by
:
This equation can be seen as Poiseuille's law with an extra correction factor expressing the average pressure relative to the outlet pressure.
Electrical circuits analogy
Electricity was originally understood to be a kind of fluid. Thishydraulic analogy
The electronic–hydraulic analogy (derisively referred to as the drain-pipe theory by Oliver Lodge) is the most widely used analogy for "electron fluid" in a metal conductor. Since electric current is invisible and the processes in play in ...
is still conceptually useful for understanding circuits. This analogy is also used to study the frequency response of fluid-mechanical networks using circuit tools, in which case the fluid network is termed a hydraulic circuit
Hydraulic machines use liquid fluid power to perform work. Heavy construction vehicles are a common example. In this type of machine, hydraulic fluid is pumped to various hydraulic motors and hydraulic cylinders throughout the machine and b ...
. Poiseuille's law corresponds to Ohm's law
Ohm's law states that the current through a conductor between two points is directly proportional to the voltage across the two points. Introducing the constant of proportionality, the resistance, one arrives at the usual mathematical equat ...
for electrical circuits, . Since the net force acting on the fluid is equal to , where , i.e. , then from Poiseuille's law, it follows that
:.
For electrical circuits, let be the concentration of free charged particles (in m−3) and let be the charge of each particle (in coulombs). (For electrons, .) Then is the number of particles in the volume , and is their total charge. This is the charge that flows through the cross section per unit time, i.e. the current
Currents, Current or The Current may refer to:
Science and technology
* Current (fluid), the flow of a liquid or a gas
** Air current, a flow of air
** Ocean current, a current in the ocean
*** Rip current, a kind of water current
** Current (stre ...
. Therefore, . Consequently, , and
:
But , where is the total charge in the volume of the tube. The volume of the tube is equal to , so the number of charged particles in this volume is equal to , and their total charge is . Since the voltage
Voltage, also known as electric pressure, electric tension, or (electric) potential difference, is the difference in electric potential between two points. In a static electric field, it corresponds to the work needed per unit of charge to m ...
, it follows then
:
This is exactly Ohm's law, where the resistance is described by the formula
:.
It follows that the resistance is proportional to the length of the resistor, which is true. However, it also follows that the resistance is inversely proportional to the fourth power of the radius , i.e. the resistance is inversely proportional to the second power of the cross section area of the resistor, which is different from the electrical formula. The electrical relation for the resistance is
:
where is the resistivity; i.e. the resistance is inversely proportional to the cross section area of the resistor. The reason why Poiseuille's law leads to a different formula for the resistance is the difference between the fluid flow and the electric current. Electron gas
An ideal Fermi gas is a state of matter which is an ensemble of many non-interacting fermions. Fermions are particles that obey Fermi–Dirac statistics, like electrons, protons, and neutrons, and, in general, particles with half-integer spin. T ...
is inviscid, so its velocity does not depend on the distance to the walls of the conductor. The resistance is due to the interaction between the flowing electrons and the atoms of the conductor. Therefore, Poiseuille's law and the hydraulic analogy
The electronic–hydraulic analogy (derisively referred to as the drain-pipe theory by Oliver Lodge) is the most widely used analogy for "electron fluid" in a metal conductor. Since electric current is invisible and the processes in play in ...
are useful only within certain limits when applied to electricity. Both Ohm's law and Poiseuille's law illustrate transport phenomena
In engineering, physics, and chemistry, the study of transport phenomena concerns the exchange of mass, energy, charge, momentum and angular momentum between observed and studied systems. While it draws from fields as diverse as continuum mechan ...
.
Medical applications – intravenous access and fluid delivery
The Hagen–Poiseuille equation is useful in determining thevascular resistance
Vascular resistance is the resistance that must be overcome to push blood through the circulatory system and create flow. The resistance offered by the systemic circulation is known as the systemic vascular resistance (SVR) or may sometimes be ca ...
and hence flow rate of intravenous (IV) fluids that may be achieved using various sizes of peripheral and central cannula
A cannula (; Latin meaning 'little reed'; plural or ) is a tube that can be inserted into the body, often for the delivery or removal of fluid or for the gathering of samples. In simple terms, a cannula can surround the inner or outer surfaces ...
s. The equation states that flow rate is proportional to the radius to the fourth power, meaning that a small increase in the internal diameter of the cannula yields a significant increase in flow rate of IV fluids. The radius of IV cannulas is typically measured in "gauge", which is to the radius. Peripheral IV cannulas are typically available as (from large to small) 14G, 16G, 18G, 20G, 22G, 26G. As an example, the flow of a 14G cannula is typically around that of a 16G, and that of a 20G. It also states that flow is inversely proportional to length, meaning that longer lines have lower flow rates. This is important to remember as in an emergency, many clinicians favor shorter, larger catheters compared to longer, narrower catheters. While of less clinical importance, an increased change in pressure () — such as by pressurizing the bag of fluid, squeezing the bag, or hanging the bag higher (relative to the level of the cannula) — can be used to speed up flow rate. It is also useful to understand that viscous fluids will flow slower (e.g. in blood transfusion
Blood transfusion is the process of transferring blood products into a person's circulation intravenously. Transfusions are used for various medical conditions to replace lost components of the blood. Early transfusions used whole blood, but mo ...
).
See also
*Couette flow
In fluid dynamics, Couette flow is the flow of a viscous fluid in the space between two surfaces, one of which is moving tangentially relative to the other. The relative motion of the surfaces imposes a shear stress on the fluid and induces flow ...
* Darcy's law
Darcy's law is an equation that describes the flow of a fluid through a porous medium. The law was formulated by Henry Darcy based on results of experiments on the flow of water through beds of sand, forming the basis of hydrogeology, a branch of e ...
* Pulse
In medicine, a pulse represents the tactile arterial palpation of the cardiac cycle (heartbeat) by trained fingertips. The pulse may be palpated in any place that allows an artery to be compressed near the surface of the body, such as at the nec ...
* Wave
In physics, mathematics, and related fields, a wave is a propagating dynamic disturbance (change from equilibrium) of one or more quantities. Waves can be periodic, in which case those quantities oscillate repeatedly about an equilibrium (res ...
* Hydraulic circuit
Hydraulic machines use liquid fluid power to perform work. Heavy construction vehicles are a common example. In this type of machine, hydraulic fluid is pumped to various hydraulic motors and hydraulic cylinders throughout the machine and b ...
Cited references
References
*. *. *.External links
Poiseuille's law for power-law non-Newtonian fluid
{{DEFAULTSORT:Hagen-Poiseuille equation Equations of fluid dynamics Mathematics in medicine